
#Derivative of log function license
Textbook content produced by OpenStax is licensed under a Creative Commons Attribution-NonCommercial-ShareAlike License 4.0 license. We recommend using aĪuthors: Gilbert Strang, Edwin “Jed” Herman Use the information below to generate a citation. Then you must include on every digital page view the following attribution: If you are redistributing all or part of this book in a digital format, Then you must include on every physical page the following attribution: If you are redistributing all or part of this book in a print format, Want to cite, share, or modify this book? This book isĬreative Commons Attribution-NonCommercial-ShareAlike License 4.0
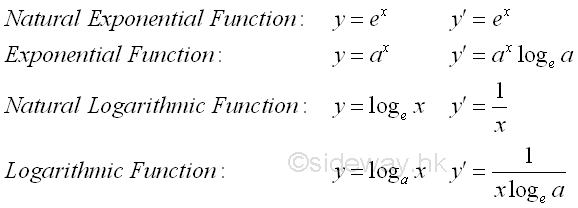
1 y d y d x = sec 2 x ln ( 2 x 4 + 1 ) + 8 x 3 2 x 4 + 1 Take the natural logarithm of both sides. d y d x = ( 2 x 4 + 1 ) tan x ( sec 2 x ln ( 2 x 4 + 1 ) + 8 x 3 2 x 4 + 1 Its inverse, L ( x ) = log e x = ln x L ( x ) = log e x = ln x is called the natural logarithmic function. The function E ( x ) = e x E ( x ) = e x is called the natural exponential function. A visual estimate of the slopes of the tangent lines to these functions at 0 provides evidence that the value of e lies somewhere between 2.7 and 2.8. Figure 3.33 provides graphs of the functions y = 2 x, y = 3 x, y = 2.7 x, y = 2 x, y = 3 x, y = 2.7 x, and y = 2.8 x. We define e e to be this unique value, as we did in Introduction to Functions and Graphs. We make one final assumption: that there is a unique value of b > 0 b > 0 for which B ′ ( 0 ) = 1. In this section, we show that by making this one additional assumption, it is possible to prove that the function B ( x ) B ( x ) is differentiable everywhere. We also assume that for B ( x ) = b x, b > 0, B ( x ) = b x, b > 0, the value B ′ ( 0 ) B ′ ( 0 ) of the derivative exists. Table 3.6 Approximating a Value of 4 π 4 π For example, we may view 4 π 4 π as the number satisfying By assuming the continuity of B ( x ) = b x, b > 0, B ( x ) = b x, b > 0, we may interpret b r b r as lim x → r b x lim x → r b x where the values of x x as we take the limit are rational. These definitions leave open the question of the value of b r b r where r r is an arbitrary real number. Later, we defined b 0 = 1, b − n = 1 b n, b 0 = 1, b − n = 1 b n, for a positive integer n, n, and b s / t = ( b t ) s b s / t = ( b t ) s for positive integers s s and t. In previous courses, the values of exponential functions for all rational numbers were defined-beginning with the definition of b n, b n, where n n is a positive integer-as the product of b b multiplied by itself n n times. The proofs that these assumptions hold are beyond the scope of this course.įirst of all, we begin with the assumption that the function B ( x ) = b x, b > 0, B ( x ) = b x, b > 0, is defined for every real number and is continuous. As we develop these formulas, we need to make certain basic assumptions. Just as when we found the derivatives of other functions, we can find the derivatives of exponential and logarithmic functions using formulas. Logarithmic functions can help rescale large quantities and are particularly helpful for rewriting complicated expressions. As we discussed in Introduction to Functions and Graphs, exponential functions play an important role in modeling population growth and the decay of radioactive materials. In this section, we explore derivatives of exponential and logarithmic functions.

#Derivative of log function how to
So far, we have learned how to differentiate a variety of functions, including trigonometric, inverse, and implicit functions.
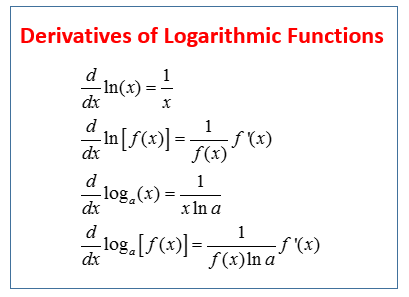
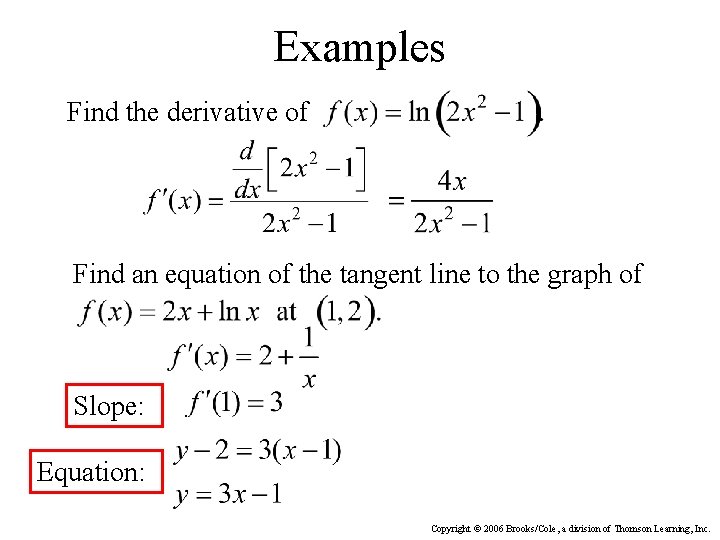
Note: if f(x) = ln x, then f '(x) = 1 / xĮxamples Example 1 Find the derivative of f(x) = log 3 xĮxample 2 Find the derivative of f(x) = ln x + 6x 2 The first derivative of f(x) = log b x is given by First Derivative of a Logarithmic Function to any Base


 0 kommentar(er)
0 kommentar(er)
